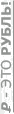NEGAPERIODIC GOLAY PAIRS AND HADAMARD MATRICES

Purpose: In analogy with the ordinary and the periodic Golay pairs, we introduce also the negaperiodic Golay pairs. (They occurred first, under a different name, in a paper of Ito.) Methods: We investigate the construction of Hadamard (and weighing) matrices from two negacyclic blocks (2N-type). The Hadamard matrices of 2N-type are equivalent to negaperiodic Golay pairs. Results: If a Hadamard matrix is also a Toeplitz matrix, we show that it must be either cyclic or negacyclic. We show that the Turyn multiplication of Golay pairs extends to a more general multiplication: one can multiply Golay pairs of length g and negaperiodic Golay pairs of length v to obtain negaperiodic Golay pairs of length gv. We show that the Ito’s conjecture about Hadamard matrices is equivalent to the conjecture that negaperiodic Golay pairs exist for all even lengths. Practical relevance: Hadamard matrices have direct practical applications to the problems of noise-immune coding and compression and masking of video information.

Вы можете купить электронную версию издания «NEGAPERIODIC GOLAY PAIRS AND HADAMARD MATRICES». После оплаты (для архивов) оно будет доступно в Личном Кабинете в разделе «Электронные издания». В случае оформления подписки, издание будет доступно по мере поступления от издателя. Формат PDF/HTML. Стоимость — от 140.00 руб.